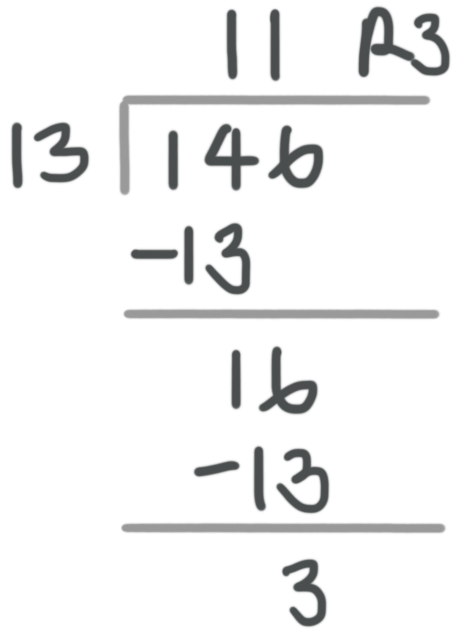Polynomial long division to simplify rational functions

Remember long division?

Do you remember doing long division? Now you probably use a calculator for most division problems. We’ll have to remember all those long division skills so that we can divide polynomials.

Think about dividing polynomials as long division, but with variables.

Krista King Math.jpg — Hi! I'm krista.

I create online courses to help you rock your math class. Read more.

Let’s review long division by dividing ???146??? by ???13???.

We start by thinking “How many times does ???13??? go into ???14????” It goes in ???1??? time , so we write a ???1??? above the long division sign and line it up with the ???4???.

Then we multiply ???13\times 1??? and get ???13???, which means we subtract ???13??? from ???14??? and get ???1???. Bring down the ???6???.

How many times does ???13??? go into ???16???? It goes in ???1??? time, so we write another ???1??? above the long division sign, this time lined up with the ???6???.

???13\times1=13???, which means we subtract ???13??? from ???16??? and get ???3???. Since ???13??? doesn’t go into ???3???, and there’s nothing left to bring down, we have a remainder of ???3???.

Our answer to ???146\div 13??? is ???11??? with a remainder of ???3???, or

???11 \frac{3}{13}???

Now let’s look at the same problem using polynomial long division. This time we’ll divide ???x^2+4x+6??? by ???x+3???.

The leading term in the dividend (???x^2+4x+6???) is ???x^2???, and the leading term in the divisor (???x+3???) is ???x???. So we start by thinking, “What do I need to multiply ???x??? by to get ???x^2????” The answer is ???x???, so we write ???x??? above the long division sign and line it up with the ???x^2???.

Then we multiply ???x+3??? by ???x??? and get ???x^2+3x???, which means we subtract ???x^2+3x??? from ???x^2+4x??? and get ???x???. Bring down the ???+6???.

What do we need to multiply ???x??? by to get ???x???? We need to multiply by ???1???, so we write ???+1??? next to the ???x??? above the long division sign.

???(x+3)\cdot1=x+3???, so we subtract ???x+3??? from ???x+6??? and get ???3???.

Our answer is ???x+1??? with a remainder of ???3???. When we do polynomial long division, we should write the remainder as a fraction, with the remainder in the numerator and the divisor in the denominator, so we should write this answer as

???x+1+\frac{3}{x+3}???

Remember to always have placeholders for any “missing” terms (terms that have a coefficient of ???0???) in the dividend. For example, if the problem above hadn’t had an ???x??? term, we would have needed to write ???x^2+0x+6??? under the long division sign.