How to find present and future value of an investment
What are the formulas for present and future value?
When we study present and future value in calculus, usually we’re trying to calculate the amount a sum of money will be worth in the future after it’s had time to grow and earn interest, or we’re trying to calculate how much money we had in the past given the sum of money in the account today.
Hi! I'm krista.
I create online courses to help you rock your math class. Read more.
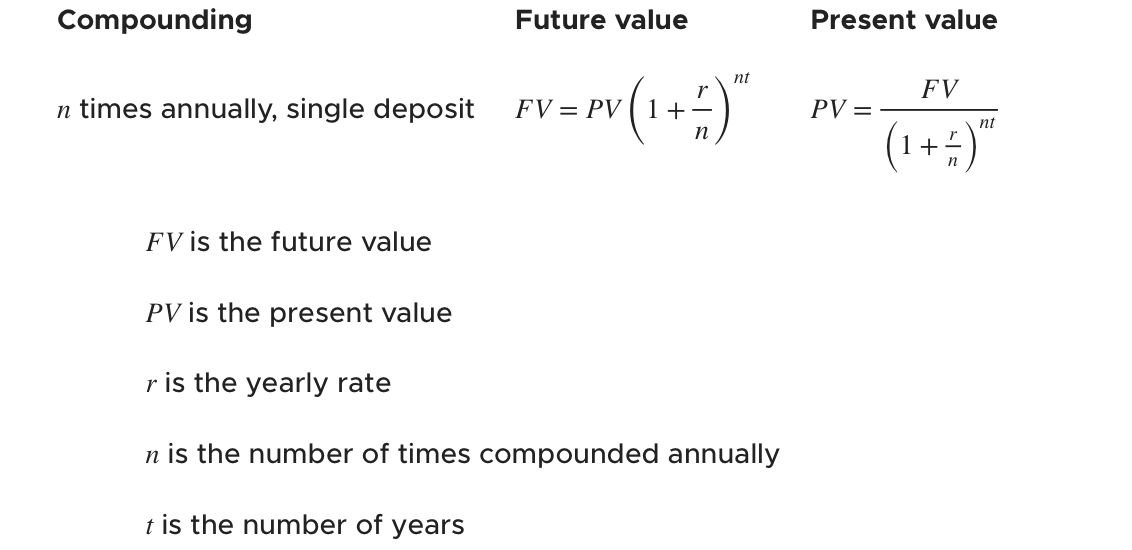
The present and future value formulas we use will vary depending on the rate at which interest is compounded, and whether we’re calculating the value of a single deposit, or a continuous income stream. Use the table below to determine which formula to use.
Finding the present value of a single deposit
Take the course
Want to learn more about Calculus 2? I have a step-by-step course for that. :)
Let’s work through several examples of how to find present and future value of an investment
Example
Find the value of a ???\$3,000??? investment after ???3??? years, if the interest rate is ???3\%??? and interest is compounded every ???3??? months (???4??? times per year).
Here’s what we know.
???PV=3,000???
???r=0.03???
???n=4???
???t=3???
Plugging these into the future value formula for interest compounded ???n??? times per year for a single deposit, we get
???FV=3,000\left(1+\frac{0.03}{4}\right)^{(4)(3)}???
???FV=3,281.42???
The value of the account after ???3??? years is ???\$ 3,281.42???.
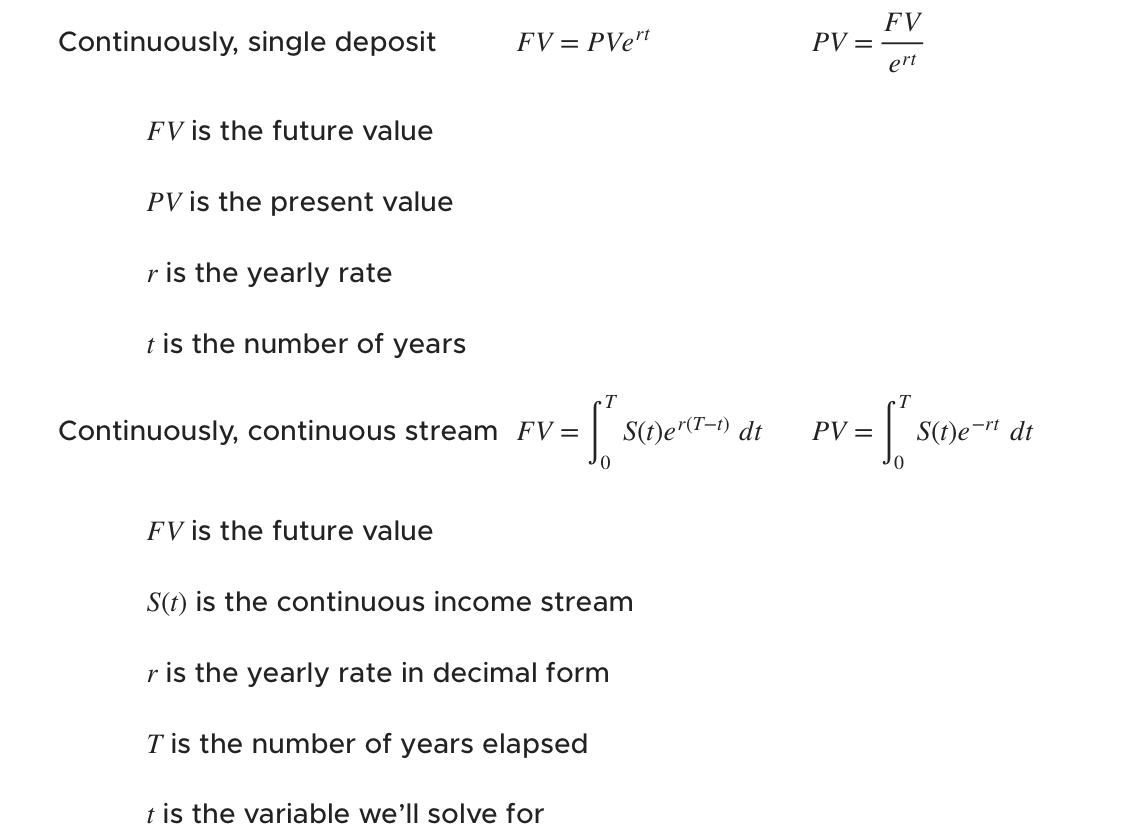
Let’s try an example in which interest is compounded continuously for a single deposit.
The present and future value formulas we use will vary depending on the rate at which interest is compounded, and whether we’re calculating the value of a single deposit, or a continuous income stream.
Example
Find the value after ???5??? years of an investment that’s worth ???\$ 1,500??? right now, if the interest rate is ???6\%??? compounded continuously.
Here’s what we know.
???PV=1,500???
???r=0.06???
???t=5??
Plugging these into the future value equation for interest compounded continuously for a single deposit, we get
???FV=1,500e^{(0.06)(5)}???
???FV=2,024.79???
The value of the account after ???5??? years is ???\$2,024.79???.
Now let’s do an example where interest is compounded continuously for a continuous income stream.
Example
Find the future value after ???3??? years of an account that has ???\$2,000??? added to it annually, if the interest rate is ???10\%??? compounded continuously. Assume that no money is withdrawn from the account during these ???3??? years, and that no money is added to the account other than the ???\$2,000??? annual deposit.
Here’s what we know.
???S(t)=2,000???
???r=0.10???
???T=3???
Plugging these into the future value equation for interest compounded continuously for a continuous income stream, we get
???FV=\int^3_0 2,000e^{0.10(3-t)}\ dt???
???FV=\int^3_0 2,000e^{0.30-0.10t}\ dt???
???FV=\int^3_0 2,000e^{0.30}e^{-0.10t}\ dt???
???FV=2,000e^{0.30}\int^3_0e^{-0.10t}\ dt???
???FV=(2,000e^{0.30})\left(\frac{e^{-0.10t}}{-0.10}\right)\bigg|^3_0???
???FV=(-20,000e^{0.30})(e^{-0.10t})\Big|^3_0???
???FV=(-20,000e^{0.30})[e^{-0.10(3)}-e^{-0.10(0)}]???
???FV=7,020.00???
The value of the account after ???3??? years is ???\$7,020.00???.
We’ll do one last example for compounding interest ???n??? times annually for a continuous income stream.
Example
You deposit ???\$10,000??? every year for ???5??? years into a new bank account. Interest on the account is compounded continuously at ???8\%???. What is the present value?
Here’s what we know.
???S(t)=10,000???
???r=0.08???
???T=5???
Plugging these into the present value equation for interest compounded ???n??? times annually for a continuous income stream, we get
???PV=\int^5_0 10,000e^{-0.08t}\ dt???
???PV=10,000\int^5_0e^{-0.08t}\ dt???
???PV=10,000\left(\frac{e^{-0.08t}}{-0.08}\right)\bigg|^5_0???
???PV=-125,000(e^{-0.08t})\Big|^5_0???
???PV=-125,000[e^{-0.08(5)}-e^{-0.08(0)}]???
???PV=41,209.99???
The value of the account today, assuming you make the ???\$10,000??? annual deposits for ???5??? years and get the interest rate you’ve been promised, is ???\$41,209.99???.