How to write word problems as equations
How words and phrases translate into math
Word problems can seem to be tricky at first. What is the problem actually asking you to do?
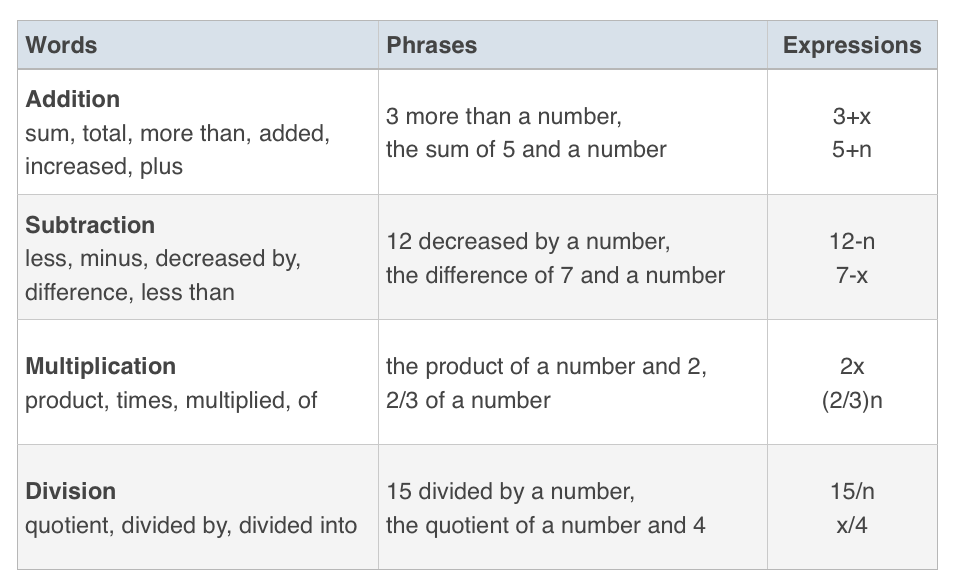
There are certain phrases that always mean the same operation in math.
The table below will help you learn common phrases in math and what operations they represent.
Hi! I'm krista.
I create online courses to help you rock your math class. Read more.
Translating words and phrases into expressions:
Examples of converting word problems into mathematical expressions and equations
Take the course
Want to learn more about Algebra 1? I have a step-by-step course for that. :)
Writing the phrase as an algebraic expression
Example
Write the phrase as an algebraic expression.
“Four less than twice ???x???”
The phrase “twice ???x???” means “???2??? times ???x???” which we know means to multiply, and so we can write it as ???2x???. Now we have
“Four less than ???2x???”
Less means subtraction, so we’ll subtract ???4??? from ???2x???.
???2x-4???
It may be tempting to write the ???4??? first and subtract ???2x???. Let’s use numbers to help us visualize this. When we say ???4??? less than ???10??? you know that you’d have to subtract ???4??? from ???10???, written out as ???10-4???. So ???4??? less than ???2x??? will be ???2x-4???.
The phrase “twice x” means “2 times x” which we know means to multiply, and so we can write it as 2x.
Example
Find the value of the expression.
???\frac{1}{4}??? of ???120???
In math, the word “of” (immediately after a proper or improper fraction) tells us to multiply. Therefore, the mathematical expression of the phrase will be
???\frac{1}{4} \cdot 120???
Because we were asked to actually find the value of the expression, we’ll perform the multiplication to get the simplified value.
???30???
Not only can we translate phrases into expressions, but we can write equations from some phrases as well.
For instance, suppose you wanted to use algebra to solve the following word problem:
John’s age is four less than twice Mary’s age. If Mary is ???18???, how old is John?
The first step in solving a word problem like this is to define the variables. What that means is to state the particular quantity that each variable stands for.
In this problem, we have two quantities: Mary’s age and John’s age. So we’ll define the variables by saying “Let ???x??? be Mary’s age, and let ???y??? be John’s age.” (We could use any letters of the alphabet for the variables, but people often use ???x??? for one of the variables, and if there are one or two additional variables, they tend to use ???y??? and ???z???, in that order.)
The next step in solving a word problem is to “translate” each word or phrase into mathematical symbols. Here, “John’s age” is translated as “???2x-4???.”
How about the word “is” (in “John’s age is four less than twice Mary’s age”)? Well, “is” is translated as an equals sign. To see this, it may help to think of the word “is” as having the same meaning (in math) as “is equal to.”
Combining all of these, we get the equation
???y=2x-4???
The third step in solving a word problem is to use the given data and solve the equation. Here, we’re given Mary’s age as ???18???, so we substitute ???18??? for ???x??? and then solve for ???y???.
???y=2(18)-4???
???y=36-4???
???y=32???
The final step is to answer the question that was asked. Here, we’re asked for John’s age. Since we defined ???y??? as John’s age, the answer is ???32???.
Suppose we’d been given the following word problem instead:
Currently, John’s age is four less than twice Mary’s age. If Mary is now ???18???, how old will John be seven years from now?
To solve this problem, it would be convenient to define ???x??? as Mary’s age now, and ???y??? as John’s age now, because we’re given a relationship between Mary’s age (now) and John’s age (now). Then in the last step (answering the question that was asked), we’d have to evaluate ???y+7??? (to get John’s age seven years from now), and our answer would be ???32+7=39???.