Factoring quadratic equations with coefficients

How to factor a trinomial with a leading coefficient

In this lesson we’ll look at methods for factoring quadratic equations with coefficients in front of the $x^2$ term (that are not $1$ or $0$).

Factoring means you’re taking the parts of an expression and rewriting it as parts that are being multiplied together (the factors). 

Factoring a quadratic equation means we will write equations of the form $ax^2+bx+c$ into the form of $(px+r)(qx+s)$, where $a,b,c,p,q,s$ are all real numbers and $a\neq 1,0$.

Hi! I'm krista.

I create online courses to help you rock your math class. Read more.

How to factor trinomials where a≠1

---

Take the course

Want to learn more about Algebra 2? I have a step-by-step course for that. :)

---

Examples of factoring quadratics with coefficients

Example

Factor the quadratic.

$3x^2+5x-2$

Let’s begin by looking at the factors of $3$ and $2$. The only factors of $3$ are $3$ and $1$, so we know we'll have 

$(3x\ \ \ \ )(x\ \ \ \ )$

The only factors of $2$ are $2$ and $1$, which means we’ll have one of the following.

$(3x \ \ 2)(x \ \ 1)$

$(3x \ \ 1)(x \ \ 2)$

Let’s see what happens if we use the first way.

$(3x \ \ 2)(x \ \ 1)=3x^2 \ \ 3x \ \ 2x \ \ 2$

We need to combine $3x$ and $2x$ in such a way that we get $5x$. But remember that in $3x^2+5x-2$, the last term $-2$ is negative, which means one of our signs has to be negative, so the only two possibilities are

$(3x+2)(x-1)=3x^2-3x+2x-2=3x^2-x-2$

$(3x-2)(x+1)=3x^2+3x-2x-2=3x^2+x-2$

But neither of these is correct because we don’t get the $+5x$ in the middle. Let’s try the second way.

$(3x \ \ 1)(x \ \ 2)=3x^2 \ \ 6x \ \ x \ \ 2$

Can we make $6x$ and $x$ into $5x$ using negative signs? Yes, we can.

$6x-x=5x$

So we finish our factors with a minus sign in front of the $1$ and a plus sign in front of the $2$.

$(3x-1)(x+2)$

---

Let’s try one more.

---

Factoring means you’re taking the parts of an expression and rewriting it as parts that are being multiplied together (the factors).

Example

Factor the quadratic.

$15x^2+66x-45$

First, we’ll factor out a $3$, because it’s a common factor between each of the three terms.

$3(5x^2+22x-15)$

Now, let’s factor $5x^2+22x-15$.

The only factors of $5$ are $5$ and $1$, so we know we’ll have 

$(5x \ \ \ \ )(x \ \ \ \ )$

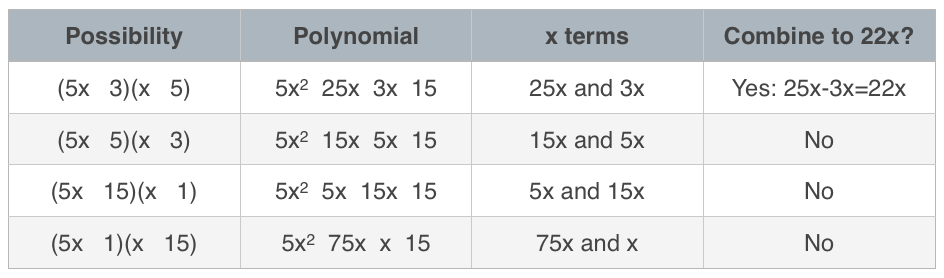
The factor pairs of $15$ are $5$ and $3$ or $1$ and $15$ so we know we’ll have one of the following.

From $5$ and $3$ we get one of two possibilities:

$(5x \ \ \ 3)(x \ \ \ 5)$

$(5x \ \ \ 5)(x \ \ \ 3)$

From $1$ and $15$ we get one of two possibilities:

$(5x \ \ \ 15)(x \ \ \ 1)$

$(5x \ \ \ 1)(x \ \ \ 15)$

Let’s look at the middle terms to see which one can give us $22x$.

Since there are so many possibilities let’s use a table to help keep them organized.

So we need to use $(5x \ \ \ 3)(x \ \ \ 5)$ and set it up to get $25x$ and $-3x$ when we multiply it out. We get $(5x-3)(x+5)$. Therefore,

$15x^2+66x-45$

$3(5x^2+22x-15)$

$3(5x-3)(x+5)$

---

Get access to the complete Algebra 2 course

---