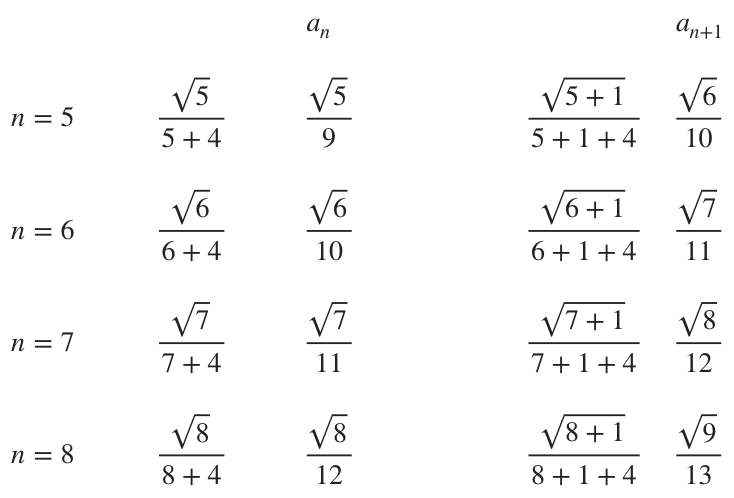How to use the alternating series test to determine convergence

What is the alternating series test?

The alternating series test for convergence lets us say whether an alternating series

$\sum^{\infty}_{n=1}(-1)^{n-1}a_n$

where $a_n>0$

is converging or diverging.

Hi! I'm krista.

I create online courses to help you rock your math class. Read more.

The alternating series test for convergence tells us that

an alternating series converges if

$0<a_{n+1}<a_n$ for all values of $n$, and

$\lim_{n\to\infty}a_n=0$

When we use the alternating series test, we need to make sure that we separate the series $a_n$ from the $(-1)^n$ part that makes it alternating.

How to use the alternating series test to determine convergence

---

Take the course

Want to learn more about Calculus 2? I have a step-by-step course for that. :)

---

Apply the alternating series test to say whether the series converges or diverges

Example

Use the alternating series test to say whether the series converges or diverges

$\sum^{\infty}_{n=5}\frac{(-1)^{n-3}\sqrt{n}}{n+4}$

First, we separate the series from the part that makes it alternating.

$\sum^{\infty}_{n=5}(-1)^{n-3}\frac{\sqrt{n}}{n+4}$

Matching this up to the standard form of an alternating series given above, we can say that the series is

$a_n=\frac{\sqrt{n}}{n+4}$

Now we need to show that

$0<a_{n+1}<a_n$ for all values of $n$, and

$\lim_{n\to\infty}a_n=0$

for the series $a_n$, in order to say that $a_n$ converges. Remembering that this series starts at $n=5$, let’s check the first few terms of the series to see if it looks like $0<a_{n+1}<a_n$.

We can see that the terms of $a_n$ and $a_{n+1}$ will always be positive, because there’s no value of $n$, when $n\geq5$, that will make either series negative. We can also see that $a_{n+1}$ is always going to be smaller than $a_n$. If you’re not convinced by their fractional values in the table, compute the decimal values on your calculator to be sure.

The alternating series test for convergence lets us say whether an alternating series is converging or diverging.

If you can’t be sure that $0<a_{n+1}<a_n$ just by looking at the table, you can always take the derivative of $a_n$ to double-check. If the derivative is negative, then you know the series is decreasing, which means that $a_{n+1}$ will always be less than $a_n$.

$\frac{d}{dx}\left(\frac{\sqrt{x}}{x+4}\right)$

Using the quotient rule, we get

$\frac{\frac12(x)^{-\frac12}(x+4)-(x)^{\frac12}(1)}{(x+4)^2}$

$\frac{\frac12x^{\frac12}+2x^{-\frac12}-x^{\frac12}}{(x+4)^2}$

$\frac{-\frac12x^{\frac12}+2x^{-\frac12}}{(x+4)^2}$

$\frac{-\frac{x^{\frac12}}{2}+\frac{2}{x^{\frac12}}}{(x+4)^2}$

$\frac{-\frac{x}{2x^{\frac12}}+\frac{4}{2x^{\frac12}}}{(x+4)^2}$

$\frac{\frac{4-x}{2x^{\frac12}}}{(x+4)^2}$

$\frac{4-x}{2x^{\frac12}}\cdot\frac{1}{(x+4)^2}$

$\frac{4-x}{2x^{\frac12}(x+4)^2}$

Looking at the derivative, we can see that for all values of the series (remember, the series starts at $n=5$), the derivative is negative because the numerator will be negative and the denominator will be positive. This confirms that the series is decreasing, and therefore that it converges.

The final step is to verify that $\lim_{n\to\infty}a_n=0$.

$\lim_{n\to\infty}a_n=\lim_{n\to\infty}\frac{\sqrt{n}}{n+4}$

$\lim_{n\to\infty}a_n=\frac{\sqrt{\infty}}{\infty+4}$

$\lim_{n\to\infty}a_n=\frac{\sqrt{\infty}}{\infty}$

Since the numerator will be significantly smaller than the denominator, especially as $n$ gets really big, we can say that

$\lim_{n\to\infty}a_n=0$

Since we’ve shown that $0<a_{n+1}<a_n$ and that $\lim_{n\to\infty}a_n=0$, we can say that the series converges.

---

Get access to the complete Calculus 2 course

---